The topic to begin every staff meeting is how are we preparing the students for the Smart Balance Assessment that correlates to the Common Core State Standards. Even to day in the department meeting our department chair was talking with us about the use of Depth of Knowledge (DoK) questions so students are accustomed to high level level questions. This evening I spent a good majority of time dissecting the practice test found at the Smarter Balance website.. This is was a good experience for me in preparation for my project in m641. In order for me to create a outstanding lesson plan I need to create an outstanding summative assessment. My goal to create a similar assessment to the one provided by the smarter balance assessment for the students at the end of their Geometry Unit that will have the Common Core State Standards as their foundation.
As I continued to take the assessment at Smarter Balance I was amazed by the number of different learning targets I was using to solve a particular problem. I was often thinking the question was written to trick the students. After continued reflection I came to the realization the assessment wanted to assess the students on a wide variety of learning objects for a single question. This is going to be a major hurtle for the way I have been teaching and for the way I was taught. So often growing up for me and seeing the released items from older MEAP assessment the questions where basic problems (i.e. add these fractions). Now they ask the students to perform a number of operations with fractions then identify if it is larger or smaller than a given value or it doesn't' ask the student to find the mean from a set of values it asks the students, "how much did the mean number of push ups increase in these two line plots from gym class."
Creating questions of this nature are going to take some time, I believe creating one unit a year would be a good goal. For this year and this class, I am going to use a majority of my last few weeks of m641 to prepare a summative assessment based on the smarter balance questions.
Monday, November 18, 2013
Tuesday, November 12, 2013
3 Different Methods to Conic Sections
As the article "Paper Folding and Conic Sections" by Scott G. Smith starts out, "One sign of a good problem can be approached in more than one way." I spent my time this week trying to achieve this goal. The first way was provided by the article by using Patti Paper, the second is hinted at by Smith but doesn't go into detail and that's by using a sketchpad, and lastly I discover on my own by having a conversation with our art teacher by creating String Art.
Paper Folding Conics:
The farthest left conic is by folding a dot off a line onto a line multiple times and its result was a parabola if we were to be done an infinite amount of times. The middle figure is a dot inside of a circle and the folding the dot onto the circle. The resulting figure when done it an infinite amount of times an ellipse. The third image is a dot outside of the circle folded onto the circle and its resulting image is a hyperbola.
As I was working on these folding sections I was thinking about what would happen if I moved the points around the circle or farther/closer to the line. I did cheat a little bit and some students after school saw me folding the paper and wanted to join, but this still only gave me a limited number of results.My next option was to work with Geogebra and see what happens.
In comparison to the Patti Paper the final image is the same. The parabola, is still created when we use the graphing software program. To create this parabola, I placed a number of points on the vertical line. Next I created bisecting lines from each point to the point off the line. I repeated the process through all the points on the vertical line. Once all the bisecting lines are gathered together you can see the shape of the parabola starting to come together.
Because I was using the Geogebra program I was able to answer some of the questions I couldn't with Patti paper. Like how the closer you get to the line the sharper the curve is of the parabola and the farther you get away the wider the parabola becomes.
 To create the ellipse I did a similar process to the parabola above. I created a circle and a point within the circle. Next I placed a number of point on the circle. Just like the project above I found the bisecting line between each point and the point outside the circle.
To create the ellipse I did a similar process to the parabola above. I created a circle and a point within the circle. Next I placed a number of point on the circle. Just like the project above I found the bisecting line between each point and the point outside the circle.
I was able to move the point inside the circle around and observe the different shapes the bisecting lines created. The closer you get to the center the closer the shape of the lines get to becoming a circle, only when the point we are moving around is the center do we get the perfect circle.

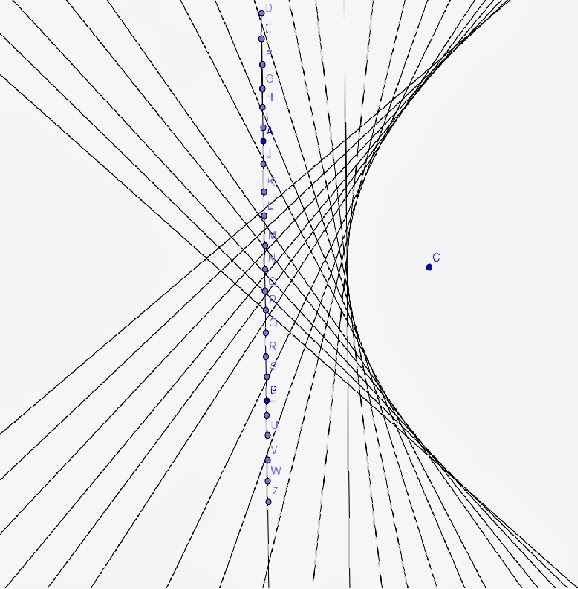
The hyperbola was created using the same program as the ellipse. The only difference is when the point is outside the circle does it turn into a hyperbola.
Using Geogebra allowed me to observe what happens when when we change the circumstances of the problem. It was very quick for me to see what happens when I place the dot I'm moving around on the line. I noticed all the lines crossed in the center of the circle. There was no true shape created by all the trend of the lines. It was one of the benefits of Geogebra. The ability to instantly move items around allow for quick feedback.
The last work with conics came to mind after a conversation at the lunch table with the art teacher. I came up with the idea after coming across the a Geoebra program were the student can design their own string project before they begin. Working with the string art was good may have been the most time consuming but it was definitely the most rewarding.
When comparing the three versions of I worked through this week, all have their highlights. If I were to judge them against another, the Geogerba program would take the cake. It is works best when it comes to manipulating the figure and seeing overall concepts. Although all would be great to work in class. I can see a future cross curricular project with the art teacher to incorporate all learners.
Paper Folding Conics:
The farthest left conic is by folding a dot off a line onto a line multiple times and its result was a parabola if we were to be done an infinite amount of times. The middle figure is a dot inside of a circle and the folding the dot onto the circle. The resulting figure when done it an infinite amount of times an ellipse. The third image is a dot outside of the circle folded onto the circle and its resulting image is a hyperbola.
As I was working on these folding sections I was thinking about what would happen if I moved the points around the circle or farther/closer to the line. I did cheat a little bit and some students after school saw me folding the paper and wanted to join, but this still only gave me a limited number of results.My next option was to work with Geogebra and see what happens.
Conics with Geogebra:
In comparison to the Patti Paper the final image is the same. The parabola, is still created when we use the graphing software program. To create this parabola, I placed a number of points on the vertical line. Next I created bisecting lines from each point to the point off the line. I repeated the process through all the points on the vertical line. Once all the bisecting lines are gathered together you can see the shape of the parabola starting to come together.
Because I was using the Geogebra program I was able to answer some of the questions I couldn't with Patti paper. Like how the closer you get to the line the sharper the curve is of the parabola and the farther you get away the wider the parabola becomes.
 To create the ellipse I did a similar process to the parabola above. I created a circle and a point within the circle. Next I placed a number of point on the circle. Just like the project above I found the bisecting line between each point and the point outside the circle.
To create the ellipse I did a similar process to the parabola above. I created a circle and a point within the circle. Next I placed a number of point on the circle. Just like the project above I found the bisecting line between each point and the point outside the circle.I was able to move the point inside the circle around and observe the different shapes the bisecting lines created. The closer you get to the center the closer the shape of the lines get to becoming a circle, only when the point we are moving around is the center do we get the perfect circle.

The hyperbola was created using the same program as the ellipse. The only difference is when the point is outside the circle does it turn into a hyperbola.
Using Geogebra allowed me to observe what happens when when we change the circumstances of the problem. It was very quick for me to see what happens when I place the dot I'm moving around on the line. I noticed all the lines crossed in the center of the circle. There was no true shape created by all the trend of the lines. It was one of the benefits of Geogebra. The ability to instantly move items around allow for quick feedback.
Conics with String Art:
The last work with conics came to mind after a conversation at the lunch table with the art teacher. I came up with the idea after coming across the a Geoebra program were the student can design their own string project before they begin. Working with the string art was good may have been the most time consuming but it was definitely the most rewarding.
When comparing the three versions of I worked through this week, all have their highlights. If I were to judge them against another, the Geogerba program would take the cake. It is works best when it comes to manipulating the figure and seeing overall concepts. Although all would be great to work in class. I can see a future cross curricular project with the art teacher to incorporate all learners.
Tuesday, November 5, 2013
Exploring Escher
I have been very fascinated with the art of M.C. Escher the last few years. His designs will captivate your mind. In our October 29th meeting of class, the professor gave us some background on the geometry behind his work. For my outside of class this week, I would do some exploration on my own. The following is my "Metacognative Memoir" or thought process, on a few of Escher's pieces of art..
My First Piece.
I started by using a "duel" technique.
Steps:
If you find the center of each of the small lizards and place a dot in the same location on each of them. For me I used the top of the back right before the the neck started to turn to the head. Once you have all the locations found, I connected each dot to its neighbor. This formed a series of triangles over the whole paper. I could tell there was a pattern starting.
For it to be "duel" you repeat the process only using the shapes your found from the first step. I took the center of each of the triangles and connected those dots to one another. When I did this last step, I used patty paper over top or the original pictire. Below is my result.
The new insight gained from these pictures gave me an appreciation for his work. I will continue to explore some additional art of M.C. Escher.
My First Piece.
I started by using a "duel" technique.
Steps:
If you find the center of each of the small lizards and place a dot in the same location on each of them. For me I used the top of the back right before the the neck started to turn to the head. Once you have all the locations found, I connected each dot to its neighbor. This formed a series of triangles over the whole paper. I could tell there was a pattern starting.
For it to be "duel" you repeat the process only using the shapes your found from the first step. I took the center of each of the triangles and connected those dots to one another. When I did this last step, I used patty paper over top or the original pictire. Below is my result.
It turns out the figure behind the lizards is an octagon and a rhombus. He used this general out line for the design of his picture.
I was very excited to see this work. With my excitement I decided to show my advanced class to students the Escher piece. There enthusiasm was greater than the experience with the Euler Line (see blog post "The Great Result"). When I showed the student the work I showed them another piece I was working on with Geogebra the same way. Geogebra of Echer's Work
This piece took me quite some time to look over. I had about three of four different trials. I don't know if this is even the right path, but it seems to work well.
Analyzing the Angels and Demons picture on Geogebra. It woks perfectly until you get to the rhombus' and then there are more dots on the paper than are used to make the figures. Also the figures are not as regular polygon as I would think they should be.
The new insight gained from these pictures gave me an appreciation for his work. I will continue to explore some additional art of M.C. Escher.
Subscribe to:
Posts (Atom)